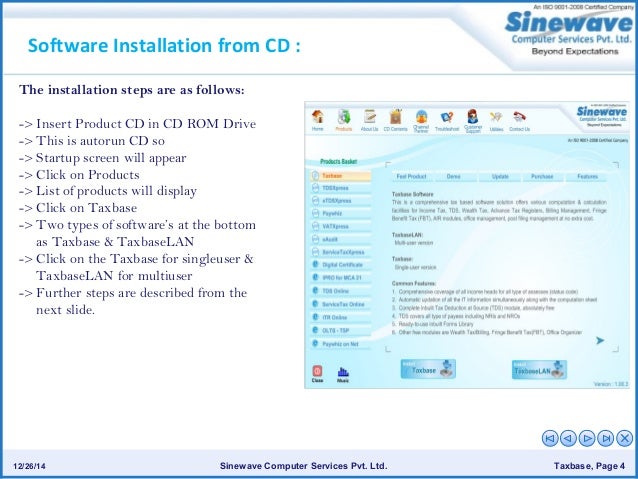
- Sinewave Taxbase Login
- Sinewave Taxbase
- Sinewave Taxbase Contact No
- Sinewave Taxbase Login
- Sinewave Taxbase Pro

To make following effect go to Miscellaneous User Parameters/Options Go to Taxbase Capital Gain untick on option Auto Determine 10% or 20% on long term capital gain on shares then save & Close After that go to Income Tax Click on Income Details Go to Capital Gain Click on New Select Type of asset Shares & Units. Taxbase GSTPro - GST Software. We have launched GST software under the name of Taxbase GSTPro. Our qualified team of Chartered Accountants and Developers keeping an eye on the updates coming from various Government sources and GSTIN in respect of technical and domain aspects of GST. Trusted Windows (PC) download Taxbase 6.2. Virus-free and 100% clean download. Get Taxbase alternative downloads.
About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How YouTube works Test new features Press Copyright Contact us Creators.
A sine wave or sinusoid is a mathematical curve that describes a smooth periodic oscillation. A sine wave is a continuous wave. It is named after the function sine, of which it is the graph. It occurs often in both pure and applied mathematics, as well as physics, engineering, signal processing and many other fields. Its most basic form as a function of time (t) is:
- y(t)=Asin(2πft+φ)=Asin(ωt+φ){displaystyle y(t)=Asin(2pi ft+varphi )=Asin(omega t+varphi )}
where:
- A, amplitude, the peak deviation of the function from zero.
- f, ordinary frequency, the number of oscillations (cycles) that occur each second of time.
- ω = 2πf, angular frequency, the rate of change of the function argument in units of radians per second
- φ{displaystyle varphi }, phase, specifies (in radians) where in its cycle the oscillation is at t = 0. When φ{displaystyle varphi } is non-zero, the entire waveform appears to be shifted in time by the amount φ{displaystyle varphi }/ω seconds. A negative value represents a delay, and a positive value represents an advance.
5 seconds of a 220 Hz sine wave. This is the sound wave described by a sine function with f = 220 oscillations per second. |
| Problems playing this file? See media help. |
The sine wave is important in physics because it retains its wave shape when added to another sine wave of the same frequency and arbitrary phase and magnitude. It is the only periodic waveform that has this property. This property leads to its importance in Fourier analysis and makes it acoustically unique.
General form[edit]
In general, the function may also have:
- a spatial variable x that represents the position on the dimension on which the wave propagates, and a characteristic parameter k called wave number (or angular wave number), which represents the proportionality between the angular frequency ω and the linear speed (speed of propagation) ν;
- a non-zero center amplitude, D
which is
- y(x,t)=Asin(kx−ωt+φ)+D{displaystyle y(x,t)=Asin(kx-omega t+varphi )+D,}, if the wave is moving to the right
- y(x,t)=Asin(kx+ωt+φ)+D{displaystyle y(x,t)=Asin(kx+omega t+varphi )+D,}, if the wave is moving to the left.
The wavenumber is related to the angular frequency by:.
- k=ωv=2πfv=2πλ{displaystyle k={omega over v}={2pi f over v}={2pi over lambda }}
where λ (lambda) is the wavelength, f is the frequency, and v is the linear speed.
This equation gives a sine wave for a single dimension; thus the generalized equation given above gives the displacement of the wave at a position x at time t along a single line.This could, for example, be considered the value of a wave along a wire.
In two or three spatial dimensions, the same equation describes a travelling plane wave if position x and wavenumber k are interpreted as vectors, and their product as a dot product. For more complex waves such as the height of a water wave in a pond after a stone has been dropped in, more complex equations are needed.
Occurrences[edit]
This wave pattern occurs often in nature, including wind waves, sound waves, and light waves.
A cosine wave is said to be sinusoidal, because cos(x)=sin(x+π/2),{displaystyle cos(x)=sin(x+pi /2),}which is also a sine wave with a phase-shift of π/2 radians. Because of this head start, it is often said that the cosine function leads the sine function or the sine lags the cosine.
The human ear can recognize single sine waves as sounding clear because sine waves are representations of a single frequency with no harmonics.
To the human ear, a sound that is made of more than one sine wave will have perceptible harmonics; addition of different sine waves results in a different waveform and thus changes the timbre of the sound. Presence of higher harmonics in addition to the fundamental causes variation in the timbre, which is the reason why the same musical note (the same frequency) played on different instruments sounds different. On the other hand, if the sound contains aperiodic waves along with sine waves (which are periodic), then the sound will be perceived to be noisy, as noise is characterized as being aperiodic or having a non-repetitive pattern.

Fourier series[edit]
Sinewave Taxbase Login
In 1822, French mathematician Joseph Fourier discovered that sinusoidal waves can be used as simple building blocks to describe and approximate any periodic waveform, including square waves. Fourier used it as an analytical tool in the study of waves and heat flow. It is frequently used in signal processing and the statistical analysis of time series.
Sinewave Taxbase
Traveling and standing waves[edit]
Since sine waves propagate without changing form in distributed linear systems,[definition needed] they are often used to analyze wave propagation. Sine waves traveling in two directions in space can be represented as
- u(t,x)=Asin(kx−ωt+φ){displaystyle u(t,x)=Asin(kx-omega t+varphi )}
Antares avox 2 review. When two waves having the same amplitude and frequency, and traveling in opposite directions, superpose each other, then a standing wave pattern is created. Note that, on a plucked string, the interfering waves are the waves reflected from the fixed end points of the string. Therefore, standing waves occur only at certain frequencies, which are referred to as resonant frequencies and are composed of a fundamental frequency and its higher harmonics. The resonant frequencies of a string are proportional to: the length between the fixed ends; the tension of the string; and inversely proportional to the mass per unit length of the string.
See also[edit]
- ∿ the sine wave symbol (U+223F)
Sinewave Taxbase Contact No
Further reading[edit]
- 'Sinusoid'. Encyclopedia of Mathematics. Springer. Retrieved December 8, 2013.
- Download
Sinewave Taxbase Login
If your download is not starting, click here.
Thank you for downloading SineWave from our software portal
The version of SineWave you are about to download is 2.0. This download is absolutely FREE. The software is periodically scanned by our antivirus system. We also encourage you to check the files with your own antivirus before launching the installation. The contents of the download are original and were not modified in any way.
SineWave antivirus report
Sinewave Taxbase Pro
This download is virus-free.This file was last analysed by Free Download Manager Lib 127 days ago.
KASPERSKY
NOD32 Nero 10 crack version free download 64-bit.
MCAFEE-GW-EDITION
WebAdvisor
To make following effect go to Miscellaneous User Parameters/Options Go to Taxbase Capital Gain untick on option Auto Determine 10% or 20% on long term capital gain on shares then save & Close After that go to Income Tax Click on Income Details Go to Capital Gain Click on New Select Type of asset Shares & Units. Taxbase GSTPro - GST Software. We have launched GST software under the name of Taxbase GSTPro. Our qualified team of Chartered Accountants and Developers keeping an eye on the updates coming from various Government sources and GSTIN in respect of technical and domain aspects of GST. Trusted Windows (PC) download Taxbase 6.2. Virus-free and 100% clean download. Get Taxbase alternative downloads.
About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How YouTube works Test new features Press Copyright Contact us Creators.
A sine wave or sinusoid is a mathematical curve that describes a smooth periodic oscillation. A sine wave is a continuous wave. It is named after the function sine, of which it is the graph. It occurs often in both pure and applied mathematics, as well as physics, engineering, signal processing and many other fields. Its most basic form as a function of time (t) is:
- y(t)=Asin(2πft+φ)=Asin(ωt+φ){displaystyle y(t)=Asin(2pi ft+varphi )=Asin(omega t+varphi )}
where:
- A, amplitude, the peak deviation of the function from zero.
- f, ordinary frequency, the number of oscillations (cycles) that occur each second of time.
- ω = 2πf, angular frequency, the rate of change of the function argument in units of radians per second
- φ{displaystyle varphi }, phase, specifies (in radians) where in its cycle the oscillation is at t = 0. When φ{displaystyle varphi } is non-zero, the entire waveform appears to be shifted in time by the amount φ{displaystyle varphi }/ω seconds. A negative value represents a delay, and a positive value represents an advance.
5 seconds of a 220 Hz sine wave. This is the sound wave described by a sine function with f = 220 oscillations per second. |
| Problems playing this file? See media help. |
The sine wave is important in physics because it retains its wave shape when added to another sine wave of the same frequency and arbitrary phase and magnitude. It is the only periodic waveform that has this property. This property leads to its importance in Fourier analysis and makes it acoustically unique.
General form[edit]
In general, the function may also have:
- a spatial variable x that represents the position on the dimension on which the wave propagates, and a characteristic parameter k called wave number (or angular wave number), which represents the proportionality between the angular frequency ω and the linear speed (speed of propagation) ν;
- a non-zero center amplitude, D
which is
- y(x,t)=Asin(kx−ωt+φ)+D{displaystyle y(x,t)=Asin(kx-omega t+varphi )+D,}, if the wave is moving to the right
- y(x,t)=Asin(kx+ωt+φ)+D{displaystyle y(x,t)=Asin(kx+omega t+varphi )+D,}, if the wave is moving to the left.
The wavenumber is related to the angular frequency by:.
- k=ωv=2πfv=2πλ{displaystyle k={omega over v}={2pi f over v}={2pi over lambda }}
where λ (lambda) is the wavelength, f is the frequency, and v is the linear speed.
This equation gives a sine wave for a single dimension; thus the generalized equation given above gives the displacement of the wave at a position x at time t along a single line.This could, for example, be considered the value of a wave along a wire.
In two or three spatial dimensions, the same equation describes a travelling plane wave if position x and wavenumber k are interpreted as vectors, and their product as a dot product. For more complex waves such as the height of a water wave in a pond after a stone has been dropped in, more complex equations are needed.
Occurrences[edit]
This wave pattern occurs often in nature, including wind waves, sound waves, and light waves.
A cosine wave is said to be sinusoidal, because cos(x)=sin(x+π/2),{displaystyle cos(x)=sin(x+pi /2),}which is also a sine wave with a phase-shift of π/2 radians. Because of this head start, it is often said that the cosine function leads the sine function or the sine lags the cosine.
The human ear can recognize single sine waves as sounding clear because sine waves are representations of a single frequency with no harmonics.
To the human ear, a sound that is made of more than one sine wave will have perceptible harmonics; addition of different sine waves results in a different waveform and thus changes the timbre of the sound. Presence of higher harmonics in addition to the fundamental causes variation in the timbre, which is the reason why the same musical note (the same frequency) played on different instruments sounds different. On the other hand, if the sound contains aperiodic waves along with sine waves (which are periodic), then the sound will be perceived to be noisy, as noise is characterized as being aperiodic or having a non-repetitive pattern.
Fourier series[edit]
Sinewave Taxbase Login
In 1822, French mathematician Joseph Fourier discovered that sinusoidal waves can be used as simple building blocks to describe and approximate any periodic waveform, including square waves. Fourier used it as an analytical tool in the study of waves and heat flow. It is frequently used in signal processing and the statistical analysis of time series.
Sinewave Taxbase
Traveling and standing waves[edit]
Since sine waves propagate without changing form in distributed linear systems,[definition needed] they are often used to analyze wave propagation. Sine waves traveling in two directions in space can be represented as
- u(t,x)=Asin(kx−ωt+φ){displaystyle u(t,x)=Asin(kx-omega t+varphi )}
Antares avox 2 review. When two waves having the same amplitude and frequency, and traveling in opposite directions, superpose each other, then a standing wave pattern is created. Note that, on a plucked string, the interfering waves are the waves reflected from the fixed end points of the string. Therefore, standing waves occur only at certain frequencies, which are referred to as resonant frequencies and are composed of a fundamental frequency and its higher harmonics. The resonant frequencies of a string are proportional to: the length between the fixed ends; the tension of the string; and inversely proportional to the mass per unit length of the string.
See also[edit]
- ∿ the sine wave symbol (U+223F)
Sinewave Taxbase Contact No
Further reading[edit]
- 'Sinusoid'. Encyclopedia of Mathematics. Springer. Retrieved December 8, 2013.
- Download
Sinewave Taxbase Login
If your download is not starting, click here.
Thank you for downloading SineWave from our software portal
The version of SineWave you are about to download is 2.0. This download is absolutely FREE. The software is periodically scanned by our antivirus system. We also encourage you to check the files with your own antivirus before launching the installation. The contents of the download are original and were not modified in any way.
SineWave antivirus report
Sinewave Taxbase Pro
This download is virus-free.This file was last analysed by Free Download Manager Lib 127 days ago.
KASPERSKY
NOD32 Nero 10 crack version free download 64-bit.
MCAFEE-GW-EDITION
WebAdvisor
Often downloaded with
- FlexiMusic Wave Editor1. FlexiMusic Wave Editor helps to open and save the audio formats namely..DOWNLOAD
- Little SineGenThe purpose of this little sine wave generator is to generate a clear sine wave..DOWNLOAD
- FlexiMusic Generator1) In FlexiMusic Generator you can generate different types of sound with..$2DOWNLOAD
- NCH Tone GeneratorTest Tone and Sound Generator Tone Generator is an easy-to-use program that..$35DOWNLOAD
- SigmaTizmSigmaTizm is a standalone MIDI-enabled virtual additive synthesizer designed..DOWNLOAD
